Understanding the perimeter of a triangle is a fundamental concept in geometry that is essential for students, engineers, architects, and anyone involved in spatial planning. Calculating the perimeter of a triangle involves summing up the lengths of its sides, which might seem straightforward at first glance, but there are nuances to consider depending on the type of triangle you're dealing with. Whether it's an equilateral, isosceles, or scalene triangle, each has its own unique properties that can affect how you approach finding the perimeter. In this comprehensive guide, we'll explore the methods and formulas necessary to accurately determine the perimeter of various triangles.
Calculating the perimeter of a triangle is not just about plugging numbers into a formula; it's about understanding the relationships between the sides and the angles of the triangle. This understanding can aid in solving more complex geometrical problems and is a skill that underpins many areas of mathematics and science. Furthermore, knowing how to find the perimeter of a triangle can be a stepping stone to mastering other geometric calculations, such as area and volume, which are crucial in multiple real-world applications.
With this knowledge, you'll be equipped to tackle a variety of problems that involve triangular shapes, whether you're designing a piece of architecture, solving a mathematical puzzle, or even planning a landscaping project. Our aim is to provide you with the tools and insights needed to approach these calculations with confidence, ensuring that you can apply these principles effectively in both academic and practical settings.
Read also:Nutritional Value Of Tapioca Balls Health Benefits And More
Table of Contents
- Basic Concepts of Triangle Perimeter
- What are the Different Types of Triangles?
- Calculating the Perimeter of an Equilateral Triangle
- How to Find the Perimeter of an Isosceles Triangle?
- Finding the Perimeter of a Scalene Triangle
- Perimeter of a Right Triangle
- Special Cases: Heron's Formula for Perimeter
- Real-World Applications of Triangle Perimeters
- Perimeter vs. Area: Understanding the Difference
- Common Mistakes in Calculating Triangle Perimeters
- Useful Tools and Resources
- A Historical Perspective of Triangle Geometry
- Frequently Asked Questions
- Conclusion
- Further Reading and Resources
Basic Concepts of Triangle Perimeter
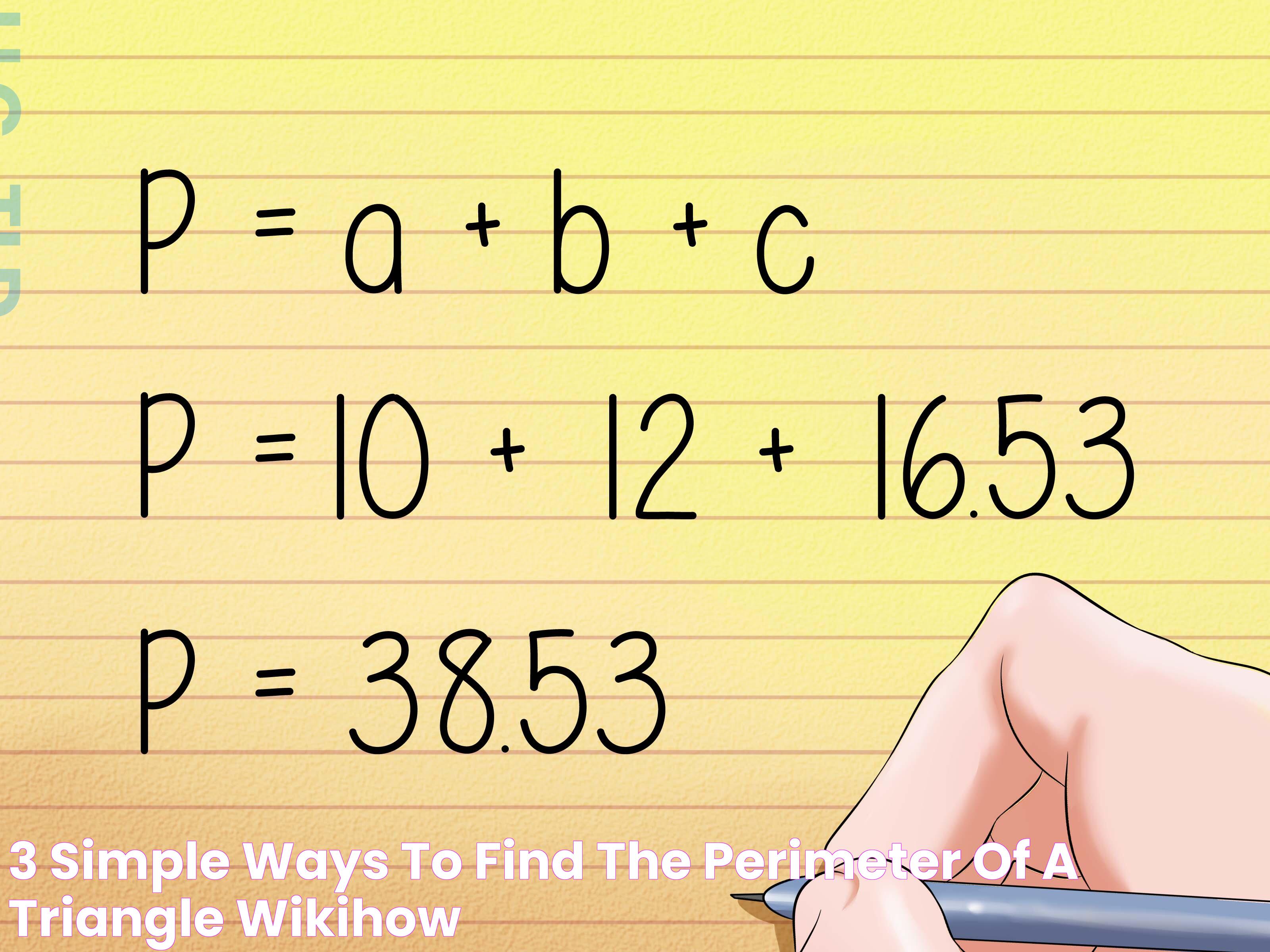
Before diving into specific calculations, let's start with the basic concept of the perimeter of a triangle. The perimeter of a triangle is the total distance around the triangle, which is the sum of the lengths of its three sides. Mathematically, this can be expressed as:
- Perimeter = a + b + c
where a, b, and c are the lengths of the sides of the triangle. This formula applies to any triangle, regardless of its type. However, the process of measuring these sides can differ based on the type of triangle, and understanding these differences is crucial for accurate calculations.
What are the Different Types of Triangles?
Triangles can be classified based on their sides and angles, and each classification has implications for calculating the perimeter. Let's take a closer look:
Classifying by Sides
- Equilateral Triangle: All three sides are equal in length.
- Isosceles Triangle: Two sides are of equal length, and the third side is different.
- Scalene Triangle: All three sides have different lengths.
Classifying by Angles
- Acute Triangle: All angles are less than 90 degrees.
- Right Triangle: One angle is exactly 90 degrees.
- Obtuse Triangle: One angle is greater than 90 degrees.
Understanding these classifications helps in applying the correct formula and method for finding the perimeter of each type of triangle.
Calculating the Perimeter of an Equilateral Triangle
An equilateral triangle is the simplest type of triangle when it comes to calculating the perimeter. Since all three sides are equal, the perimeter can be easily calculated using the formula:
- Perimeter = 3 × side_length
For example, if each side of an equilateral triangle is 5 cm, the perimeter would be:
Read also:Mastering The Art Of Selling Domain Names On Godaddy A Stepbystep Guide
- Perimeter = 3 × 5 = 15 cm
This simplicity makes equilateral triangles a favorite among educators when introducing concepts of perimeter to students.
How to Find the Perimeter of an Isosceles Triangle?
Isosceles triangles have two sides of equal length, which influences how we calculate the perimeter. The formula for the perimeter of an isosceles triangle is:
- Perimeter = 2 × side_length + base_length
For instance, if the two equal sides of an isosceles triangle measure 7 cm each, and the base measures 5 cm, the perimeter would be:
- Perimeter = 2 × 7 + 5 = 19 cm
This formula highlights the importance of accurately measuring all sides, especially the base, which distinguishes it from an equilateral triangle.
Finding the Perimeter of a Scalene Triangle
The scalene triangle, with all sides of different lengths, requires that each side be measured individually. The perimeter is calculated using the standard formula:
- Perimeter = a + b + c
Consider a scalene triangle with sides measuring 6 cm, 8 cm, and 10 cm. The perimeter is calculated as follows:
- Perimeter = 6 + 8 + 10 = 24 cm
It's crucial to ensure that each measurement is accurate, as the uniqueness of each side means there is no room for error or assumption in calculations.
Perimeter of a Right Triangle
A right triangle is a special type of scalene triangle where one angle measures 90 degrees. This introduces the Pythagorean Theorem, which can be used to find the length of the hypotenuse if the other two sides are known:
- Hypotenuse (c) = √(a² + b²)
Once the hypotenuse is determined, the perimeter can be calculated as:
- Perimeter = a + b + c
For example, in a right triangle with sides 3 cm and 4 cm, the hypotenuse would be:
- c = √(3² + 4²) = √(9 + 16) = √25 = 5 cm
- Perimeter = 3 + 4 + 5 = 12 cm
This approach demonstrates the interplay between different mathematical concepts, such as geometry and algebra, in finding the perimeter.
Special Cases: Heron's Formula for Perimeter
Heron's formula is a powerful tool for calculating the perimeter of a triangle when only the lengths of the sides are known. It's particularly useful in complex geometrical problems where angles are not readily given. The formula is expressed as:
- s = (a + b + c) / 2
- Area = √[s × (s - a) × (s - b) × (s - c)]
Although primarily used for finding the area, understanding the semi-perimeter (s) gives an intermediate step that emphasizes the perimeter's role in further calculations.
Real-World Applications of Triangle Perimeters
The concept of perimeter extends beyond theoretical exercises and finds application in various real-world scenarios. Here are a few examples:
- Architecture: Determining the perimeter is crucial in planning the layout of triangular plots or structures.
- Landscaping: Triangle perimeters help in outlining gardens, walkways, and patios.
- Navigation: In navigation, perimeter calculations assist in plotting courses and determining distances.
These applications show the practical importance of understanding how to find the perimeter of a triangle, as it assists in making informed decisions in multiple fields.
Perimeter vs. Area: Understanding the Difference
While both perimeter and area are fundamental geometric concepts, they serve different purposes. The perimeter measures the distance around a shape, whereas the area measures the space within it. This distinction is crucial in various applications:
- Perimeter: Used in fencing, border design, and track lengths.
- Area: Essential for calculating floor space, material usage, and land area.
Understanding the difference between these concepts helps in applying them correctly depending on the problem at hand.
Common Mistakes in Calculating Triangle Perimeters
Despite its straightforward nature, calculating the perimeter of a triangle can lead to errors if certain pitfalls are not avoided:
- Incorrect Measurements: Ensure all sides are measured accurately to avoid incorrect calculations.
- Confusing Area with Perimeter: Remember, perimeter is the sum of the sides, not the space enclosed.
- Misapplying Formulas: Use the correct formula for the specific type of triangle you're working with.
Avoiding these common mistakes will lead to more accurate and reliable results in your calculations.
Useful Tools and Resources
Several tools and resources can assist in calculating the perimeter of a triangle more efficiently:
- Online Calculators: Websites like Calculator.net offer useful tools for quick calculations.
- Geometry Software: Programs like GeoGebra provide visual aids and interactive calculations.
- Educational Videos: Platforms like Khan Academy offer tutorials on triangle calculations.
These resources can enhance your understanding and make the process of finding the perimeter more accessible.
A Historical Perspective of Triangle Geometry
The study of triangles dates back to ancient civilizations, where it played a crucial role in the development of mathematics. From the pyramids of Egypt to the navigational systems of the Greeks, understanding triangle geometry has been fundamental. Over time, these principles have evolved, influencing modern geometry and mathematics education.
Frequently Asked Questions
What is the formula for the perimeter of a triangle?
The formula for the perimeter of a triangle is the sum of the lengths of its sides: Perimeter = a + b + c.
Can the perimeter of a triangle be calculated with only two sides known?
No, you need the lengths of all three sides to calculate the perimeter of a triangle accurately.
How does the perimeter of a triangle differ from its area?
The perimeter measures the total distance around the triangle, while the area measures the space enclosed within the triangle.
Why is it important to learn how to find the perimeter of a triangle?
Understanding how to find the perimeter is essential for solving various geometric problems and has practical applications in fields like architecture and engineering.
What tools can help in calculating the perimeter of a triangle?
Online calculators, geometry software, and educational videos are useful tools for calculating and understanding triangle perimeters.
What are some applications of triangle perimeters in real life?
Triangle perimeters are used in architecture, landscaping, and navigation for planning and designing spaces and routes.
Conclusion
Understanding how to find the perimeter of a triangle is a foundational skill in geometry that applies to various real-world situations. By mastering the formulas and methods discussed, you'll be well-equipped to tackle a broad range of problems involving triangles. Whether you're a student, professional, or enthusiast, this knowledge can enhance your mathematical abilities and open up new possibilities for application.
Further Reading and Resources
To deepen your understanding of triangle geometry, consider exploring more resources on topics such as Heron's Formula and the Pythagorean Theorem. Websites like Math is Fun offer additional insights and exercises to practice your skills.