In the realm of mathematics and geometry, understanding how to get tangent is an essential skill that forms the backbone of many advanced concepts and applications. The tangent, a line that touches a curve at a single point without crossing it, plays a critical role in calculus, trigonometry, and various fields of science and engineering. As students and professionals delve deeper into these subjects, acquiring a robust grasp of tangent lines and their properties becomes increasingly important.
For many, the journey to mastering how to get tangent begins with a solid foundation in basic mathematical principles, followed by the exploration of more complex geometrical relationships. The tangent is not only a key concept in mathematics but also a gateway to understanding more complicated theorems and problems. Whether it's calculating angles in a circle or determining the slope at a particular point on a curve, the knowledge of how to get tangent proves invaluable in a multitude of scenarios.
This comprehensive guide aims to provide readers with a thorough understanding of how to get tangent, offering insights into its various applications, methods of calculation, and practical tips for mastering this fundamental concept. By the end of this article, readers will be equipped with the knowledge and tools necessary to tackle tangent-related problems with confidence and precision.
Read also:Mastering Maps Google Directions Driving Your Ultimate Guide
Table of Contents
- Understanding Tangent
- Why is Tangent Important?
- Basic Concepts of Tangent
- What are the Practical Applications of Tangent?
- How to Calculate Tangent?
- Tangent in Trigonometry
- The Role of Tangent in Calculus
- How to Use Tangent in Real-Life Situations?
- Common Mistakes When Finding Tangent
- How to Improve Your Tangent Skills?
- Frequently Asked Questions
- Conclusion
Understanding Tangent
A tangent is a line that touches a curve at a single point without crossing it. In simpler terms, it's the line that just 'grazes' the curve. The concept of the tangent is fundamental in calculus, where it is used to find the slope of a curve at a particular point. This slope represents the rate of change of the curve, which is crucial in understanding the behavior of functions.
The tangent line's properties are dictated by the curve it touches. For instance, in a circle, the tangent at any point is perpendicular to the radius at that point. In terms of equations, if a curve is represented by a function y = f(x), then the equation of the tangent at a point (a, f(a)) can be found using the derivative f'(a), which gives the slope of the tangent line.
Properties of Tangent Lines
- The tangent touches the curve at only one point.
- The tangent is perpendicular to the radius of a circle at the point of tangency.
- The slope of the tangent at a point on a curve is given by the derivative at that point.
Historical Context of Tangent
The concept of the tangent has been around since ancient times. The Greeks, particularly, used tangents in their geometric studies. The word "tangent" comes from the Latin "tangens," meaning "touching." Over the centuries, mathematicians have developed the concept further, applying it to various fields and problems.
Why is Tangent Important?
The importance of understanding how to get tangent cannot be overstated. Tangents are crucial in various mathematical and scientific applications. They help in understanding the rate of change, which is a fundamental concept in calculus. Tangents are also used in physics to describe motion, in engineering for designing curves, and in computer graphics for rendering curves and surfaces.
One of the main reasons tangents are essential is their role in derivatives. In calculus, the derivative of a function at a point is the slope of the tangent line at that point. This concept provides insight into the behavior of functions, helping us understand how they change and predict future values.
Applications in Science and Engineering
In engineering, tangents are used to design roads, bridges, and buildings. They help in calculating the optimal angles and slopes, ensuring structures are safe and efficient. In computer graphics, tangents are used to render curves smoothly, providing realistic images and animations.
Read also:Two Roads Diverge In A Yellow Wood An Indepth Analysis
Role in Mathematical Theorems
In mathematics, tangents are used in various theorems and proofs. For instance, the Mean Value Theorem in calculus relies on the concept of the tangent to prove that a continuous function has at least one point where the tangent is parallel to the secant line.
Basic Concepts of Tangent
Before diving into advanced applications, it's essential to grasp the basic concepts related to how to get tangent. At its core, a tangent line is defined by its point of tangency and its slope. Understanding these basic elements is crucial for working with tangents in more complex scenarios.
The point of tangency is where the tangent touches the curve. This point is critical because it determines the tangent's position and orientation. The slope of the tangent, on the other hand, indicates the rate at which the curve is changing at that specific point. This slope is calculated using derivatives, which are foundational in calculus.
Calculating the Slope of a Tangent
The slope of a tangent line is calculated using the derivative of the function that defines the curve. If a function is given as y = f(x), the slope of the tangent at a point x = a is given by the derivative f'(a). This derivative represents the instantaneous rate of change of the function at that point.
Tangent and Normal Lines
In geometry, the tangent at a point on a curve is related to the normal line. The normal line is perpendicular to the tangent at the point of tangency. Understanding the relationship between tangents and normals is important in various applications, including physics and engineering.
What are the Practical Applications of Tangent?
The practical applications of understanding how to get tangent extend across various fields, including science, engineering, and computer graphics. In each of these areas, tangents provide essential insights and solutions to complex problems.
Engineering and Design
In engineering, tangents are used extensively in design and construction. For example, when designing roads and bridges, engineers need to calculate the optimal angles and slopes to ensure safety and efficiency. Tangents help in determining these critical parameters, enabling the construction of safe and reliable structures.
Physics and Motion
In physics, tangents are used to describe motion. The tangent to a trajectory at a point provides the direction of the velocity of an object. This information is crucial in understanding the motion of objects and predicting their future positions.
Computer Graphics and Animation
In computer graphics, tangents are used to render curves and surfaces smoothly. By calculating the tangents at various points on a curve, computer programs can generate realistic images and animations. This application is particularly important in video games and movies, where realism is paramount.
How to Calculate Tangent?
Calculating the tangent of a curve involves finding the equation of the tangent line at a particular point. This process requires knowledge of calculus, specifically derivatives, which provide the slope of the tangent at the point of interest.
Steps to Calculate Tangent
- Identify the function that defines the curve.
- Determine the point at which you want to find the tangent.
- Calculate the derivative of the function to find the slope of the tangent.
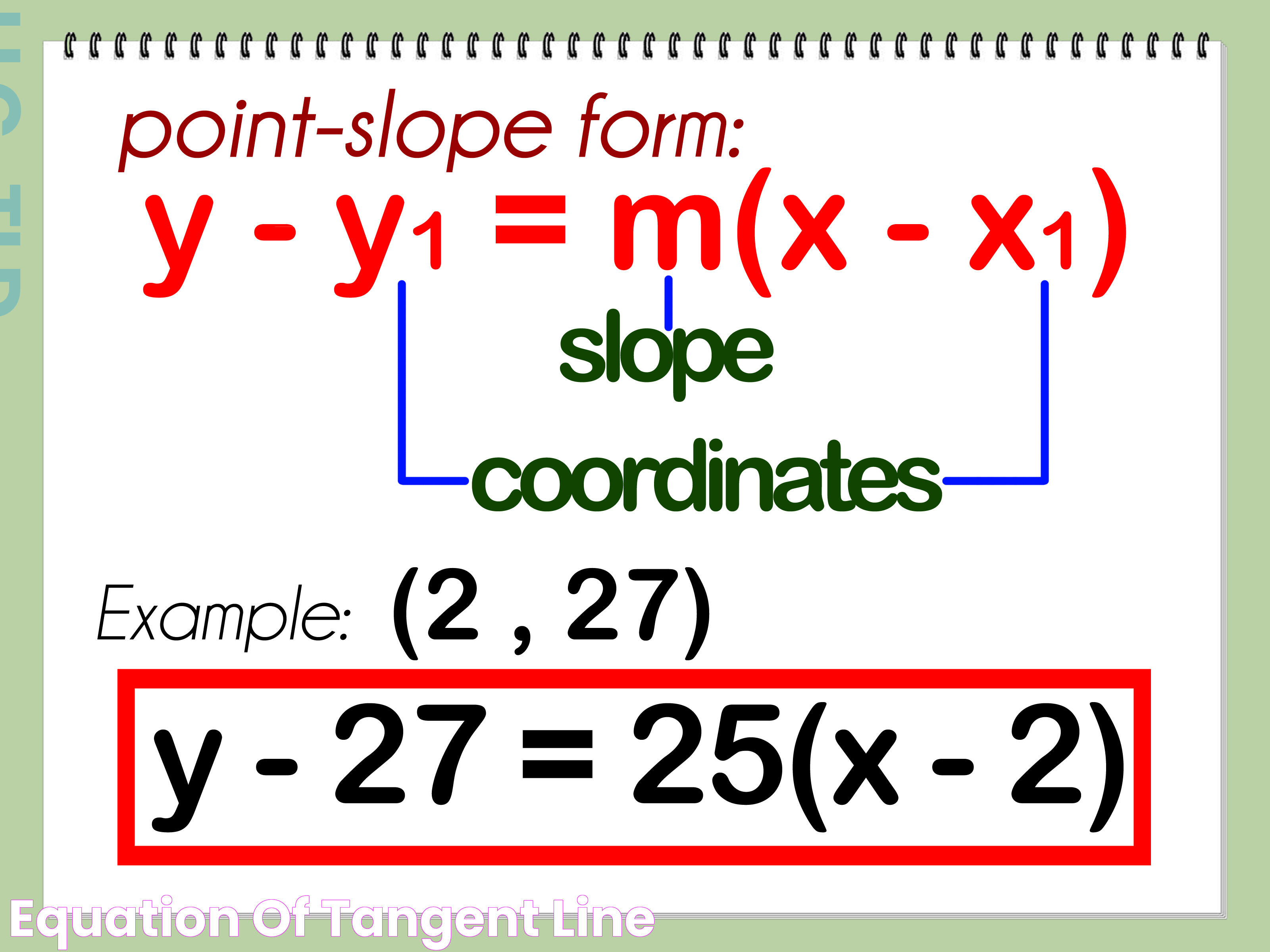
- Use the point-slope form of a line to find the equation of the tangent.
Example Calculation
Consider the function y = x^2. To find the tangent at the point (1, 1), we first calculate the derivative, which is 2x. At x = 1, the slope of the tangent is 2. Using the point-slope form, the equation of the tangent is y - 1 = 2(x - 1), which simplifies to y = 2x - 1.
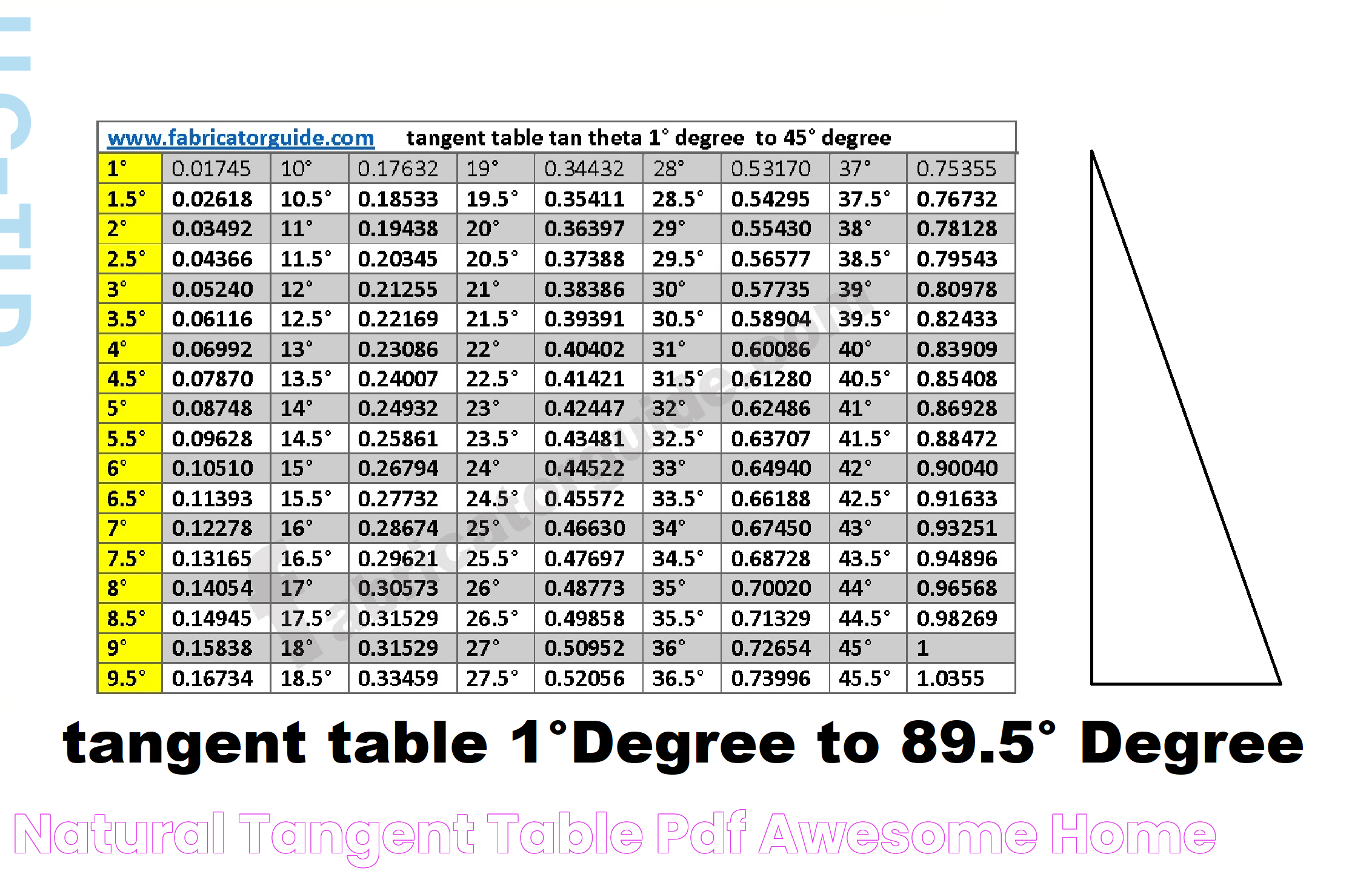
Tangent in Trigonometry
In trigonometry, the tangent of an angle is a fundamental concept that relates the sides of a right triangle. It is defined as the ratio of the opposite side to the adjacent side. Understanding this concept is crucial for solving trigonometric problems and applications.
Trigonometric Functions and Tangent
The tangent function is one of the primary trigonometric functions, alongside sine and cosine. It is used to calculate angles and distances in various applications, including navigation, astronomy, and architecture.
Using Tangent in Right Triangles
In a right triangle, the tangent of an angle θ is given by the formula:
tan(θ) = opposite / adjacent
This formula is used to solve for unknown sides and angles in right triangles, making it a valuable tool in geometry and trigonometry.
The Role of Tangent in Calculus
In calculus, the concept of the tangent is central to understanding derivatives and integrals. The tangent line's slope at a point on a curve provides the derivative, which is a measure of the function's rate of change at that point.
Derivatives are used in various applications, including optimization problems, motion analysis, and curve sketching. Understanding how to get tangent is crucial for solving these problems and gaining insights into the behavior of functions.
The Derivative as a Tangent Slope
The derivative of a function at a point is defined as the slope of the tangent line at that point. This concept is fundamental in calculus, where derivatives are used to analyze and predict the behavior of functions.
Applications of Tangents in Calculus
In calculus, tangents are used in various applications, including:
- Finding maximum and minimum points of functions.
- Analyzing the concavity and inflection points of curves.
- Solving differential equations.
How to Use Tangent in Real-Life Situations?
Understanding how to get tangent is not only useful in academic settings but also in various real-life situations. From calculating slopes in construction projects to determining angles in navigation, tangents play a crucial role in solving practical problems.
Construction and Architecture
In construction and architecture, tangents are used to calculate slopes and angles, ensuring structures are stable and aesthetically pleasing. Architects and engineers rely on tangents to design buildings, bridges, and roads with precision.
Navigation and Surveying
In navigation and surveying, tangents help in determining angles and distances. Surveyors use tangents to measure land and create accurate maps, while navigators use them to chart courses and determine positions.
Everyday Applications
In everyday life, tangents are used in various tasks, such as calculating the slope of a ramp or the angle of a roof. Understanding how to get tangent can simplify these tasks and provide accurate results.
Common Mistakes When Finding Tangent
While calculating tangents is a straightforward process, there are common mistakes that learners often make. Being aware of these mistakes can help you avoid them and improve your understanding of how to get tangent.
Misidentifying the Point of Tangency
One common mistake is misidentifying the point of tangency. Ensure you have correctly identified the point where the tangent touches the curve, as this determines the slope and equation of the tangent.
Incorrect Derivative Calculation
Another mistake is incorrect derivative calculation. Make sure you have calculated the derivative accurately, as this provides the slope of the tangent. Double-check your calculations to avoid errors.
Confusing Tangent with Secant
It's also easy to confuse the tangent with the secant, which is a line that intersects a curve at two points. Remember that a tangent only touches the curve at one point.
How to Improve Your Tangent Skills?
Improving your skills in understanding how to get tangent requires practice and a clear understanding of the concept. Here are some tips to enhance your skills:
Practice Regularly
Regular practice is key to mastering tangents. Solve a variety of problems, from basic to advanced, to strengthen your understanding and application of tangents.
Review Mathematical Concepts
Make sure you have a strong grasp of the foundational mathematical concepts, such as derivatives and slopes, as these are essential for understanding tangents.
Utilize Online Resources
Take advantage of online resources, such as tutorials and practice problems, to reinforce your learning. Websites like Khan Academy and Wolfram Alpha offer valuable tools and information on tangents.
Frequently Asked Questions
What is a tangent line?
A tangent line is a straight line that touches a curve at a single point without crossing it. It represents the instantaneous rate of change of the curve at that point.
How do you calculate the tangent to a curve?
To calculate the tangent to a curve, find the derivative of the function defining the curve to determine the slope at the point of tangency. Use this slope in the point-slope form of a line to find the equation of the tangent.
What is the difference between a tangent and a secant?
A tangent touches a curve at one point, while a secant intersects a curve at two points. The tangent represents the instantaneous rate of change, while the secant represents the average rate of change between two points.
Why is the tangent important in calculus?
The tangent is important in calculus because it provides the derivative, which is a measure of the rate of change of a function. Derivatives are used to analyze and predict the behavior of functions.
Can tangents be used in real-life applications?
Yes, tangents have numerous real-life applications, including in engineering, physics, and computer graphics. They help in calculating slopes, angles, and rates of change.
How can I improve my understanding of tangents?
To improve your understanding of tangents, practice regularly, review mathematical concepts, and utilize online resources. Solving a variety of problems will enhance your skills and confidence.
Conclusion
Understanding how to get tangent is an invaluable skill with wide-ranging applications in mathematics, science, engineering, and everyday life. By grasping the fundamental principles and practicing regularly, you can master this concept and apply it to solve complex problems. Whether you're calculating slopes in construction or rendering curves in computer graphics, the knowledge of tangents opens up a world of possibilities and insights. Keep exploring and practicing, and you'll find yourself well-equipped to tackle any tangent-related challenge that comes your way.